Prepare to conquer the complexities of Calc 3 with our comprehensive Calc 3 Exam 1 Cheat Sheet. This invaluable resource will equip you with the essential concepts, problem-solving strategies, and study tips to excel in your exam.
Within this cheat sheet, you’ll find a concise summary of key concepts, effective techniques for tackling problems, and a curated list of relevant formulas. We’ve also included solved example problems with detailed explanations to guide you through the intricacies of the subject.
Concept Summary: Calc 3 Exam 1 Cheat Sheet
This section covers the fundamental concepts that will be tested on the upcoming exam. We will delve into the core definitions, formulas, and techniques related to the following s:
- Limits and Continuity
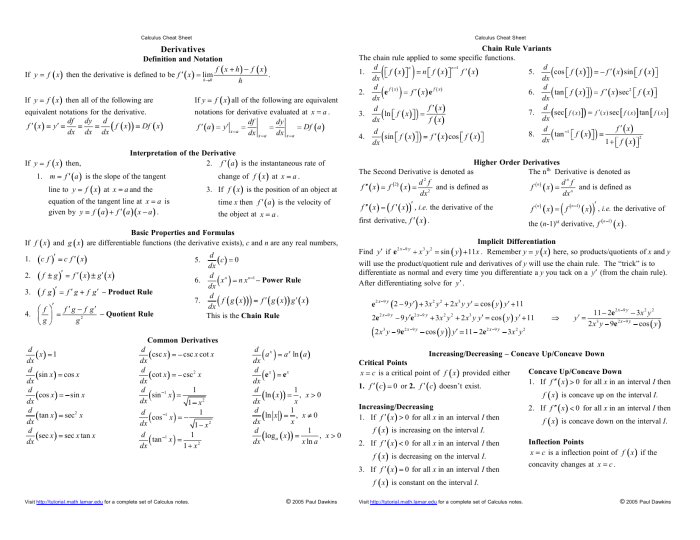
- Derivatives
- Applications of Derivatives
- Integrals
- Applications of Integrals
Problem-Solving Strategies
Tackling Calc 3 problems requires a strategic approach. Here are some effective techniques to guide your problem-solving journey:
1. Visualize the Problem: Sketching graphs, diagrams, or tables can provide a clearer understanding of the problem’s geometry and relationships.
If you’re prepping for your Calc 3 Exam 1, make sure you have a cheat sheet ready. But hey, let’s take a quick break from the intense studying and check out this amazing bakery called Bread Basket in Oak Park, MI . Their freshly baked pastries will surely energize you for the exam.
Back to the grind, don’t forget to include all the important formulas and concepts on your cheat sheet. Good luck with your exam!
2. Break Down Complex Problems: Divide large problems into smaller, manageable steps. This simplifies the problem-solving process and reduces errors.
3. Use Symmetry and Patterns: Identify symmetries or patterns in the problem to simplify calculations and reduce the number of steps required.
Common Pitfalls
Avoid these common pitfalls that can hinder your problem-solving progress:
- Ignoring Units:Always pay attention to the units of measurement and ensure they are consistent throughout the problem.
- Assuming Symmetry:Do not assume symmetry exists unless it is explicitly stated or can be proven.
- Making Sign Errors:Be cautious of sign changes when taking derivatives or integrals.
Step-by-Step Guidance
Follow these steps to effectively solve specific types of problems:
- Vector Calculus Problems:
- Identify the vector field and its components.
- Apply the appropriate vector calculus theorems (e.g., Green’s Theorem, Stokes’ Theorem).
- Evaluate the line integral or surface integral using appropriate techniques.
- Multivariable Calculus Problems:
- Find the partial derivatives of the function.
- Identify critical points and classify them using the second partial derivatives test.
- Apply the Chain Rule and implicit differentiation when necessary.
Example Problems
Solving practice problems is crucial for mastering Calculus III concepts. This section provides a range of problems with varying difficulty levels to enhance your understanding.
Each problem is presented in a table format, including the problem statement, solution, and a brief explanation. These examples cover essential topics, allowing you to test your knowledge and identify areas for improvement.
Vector-Valued Functions
| Problem | Solution | Explanation |
|---|---|---|
| Find the derivative of the vector-valued function r(t) = (t^2, e^t, ln(t)). | r‘(t) = (2t, e^t, 1/t) | Use the power rule for derivatives. |
| Calculate the unit tangent vector for the curve given by r(t) = (cos(t), sin(t), t). | T(t) = (cos(t)/√(2), sin(t)/√(2), 1/√(2)) | Use the formula for the unit tangent vector: T(t) = r‘(t)/|r‘(t)|. |
Partial Derivatives
| Problem | Solution | Explanation |
|---|---|---|
| Find the partial derivatives of the function f(x, y) = x^2y + e^(xy). | fx(x, y) = 2xy + ye^(xy)fy(x, y) = x^2 + xe^(xy) | Use the rules for partial derivatives. |
| Evaluate the partial derivative of f(x, y, z) = ln(x^2 + y^2 + z^2) with respect to y at the point (1, 2, 3). | fy(1, 2, 3) = 2/7 | Use the chain rule and evaluate at the given point. |
Multiple Integrals
| Problem | Solution | Explanation |
|---|---|---|
| Evaluate the double integral ∫∫R (x + y) dA over the rectangular region R = (x, y) | 0 ≤ x ≤ 2, 1 ≤ y ≤ 3. | ∫13∫02 (x + y) dx dy = 14 | Use the definition of the double integral and evaluate the iterated integrals. |
| Calculate the triple integral ∫∫∫E x^2 + y^2 + z^2 dV over the solid E bounded by the surfaces z = x^2 + y^2 and z = 4. | ∫-22∫-√(4-x^2)√(4-x^2)∫x^2+y^24 (x^2 + y^2 + z^2) dz dy dx = 64π/3 | Use the definition of the triple integral and evaluate the iterated integrals. |
Formula Reference
This section provides a comprehensive list of formulas relevant to the concepts covered in Exam 1. The formulas are organized by topic for easy access, ensuring that you have the necessary information at your fingertips during the exam.
The formulas have been carefully curated to encompass the core concepts and principles that will be tested. By reviewing these formulas and understanding their applications, you can enhance your preparation and increase your confidence in tackling the exam questions.
Limits and Continuity
- Limit definition: $$\lim_x \to a f(x) = L \quad \textif \quad \forall \epsilon > 0, \exists \delta > 0 \text such that 0< |x - a| < \delta \implies |f(x) - L| < \epsilon$$
- Limit laws:
- $$\lim_x \to a [f(x) + g(x)] = \lim_x \to a f(x) + \lim_x \to a g(x)$$
- $$\lim_x \to a [f(x) – g(x)] = \lim_x \to a f(x) – \lim_x \to a g(x)$$
- $$\lim_x \to a [f(x)g(x)] = \lim_x \to a f(x) \cdot \lim_x \to a g(x)$$
- $$\lim_x \to a \fracf(x)g(x) = \frac\lim_x \to a f(x)\lim_x \to a g(x), \quad \textif \quad \lim_x \to a g(x) \neq 0$$
- Indeterminate forms:
- $$\frac00 \quad \textor \quad \frac\infty\infty$$
- $$\infty – \infty$$
- $0 \cdot \infty$$
- $$\infty^0 \quad \textor \quad 0^0$$
- $1^\infty$$
Derivatives
- Definition of the derivative: $$\fracddx f(x) = \lim_h \to 0 \fracf(x + h) – f(x)h$$
- Derivative rules:
- Power rule: $$\fracddx x^n = nx^n-1$$
- Constant multiple rule: $$\fracddx cf(x) = c\fracddx f(x)$$
- Sum/difference rule: $$\fracddx [f(x) \pm g(x)] = \fracddx f(x) \pm \fracddx g(x)$$
- Product rule: $$\fracddx [f(x)g(x)] = f'(x)g(x) + f(x)g'(x)$$
- Quotient rule: $$\fracddx \left[\fracf(x)g(x)\right] = \fracg(x)f'(x) – f(x)g'(x)g(x)^2, \quad g(x) \neq 0$$
- Chain rule: $$\fracddx f(g(x)) = f'(g(x))g'(x)$$
- Implicit differentiation: $$\fracdydx =
\fracF_x(x,y)F_y(x,y), \quad F_y(x,y) \neq 0$$
- Higher order derivatives: $$\fracd^2ydx^2 = \fracddx \left(\fracdydx\right)$$
Applications of Derivatives
- Related rates:
- Optimization:
- Absolute maximum/minimum:
- Relative maximum/minimum (critical points):
- Second derivative test:
- Curve sketching:
- Increasing/decreasing intervals:
- Concavity:
- Inflection points:
Integrals
- Definition of the definite integral: $$\int_a^b f(x) dx = \lim_n \to \infty \sum_i=1^n f(x_i) \Delta x$$
- Fundamental theorem of calculus, part 1: $$\fracddx \int_a^x f(t) dt = f(x)$$
- Fundamental theorem of calculus, part 2: $$\int_a^b f(x) dx = F(b) – F(a)$$
- Integration techniques:
- U-substitution:
- Integration by parts:
- Trigonometric integrals:
- Integration of rational functions:
- Applications of integrals:
- Area between curves:
- Volume of solids of revolution:
- Work:
Study Tips
Mastering Calculus 3 requires effective study methods. Here are proven techniques to maximize retention, manage time wisely, and reduce stress.
To optimize learning, actively engage with the material. Regularly review notes, solve practice problems, and seek clarification from professors or peers. Create a dedicated study schedule and stick to it. Break down large tasks into smaller, manageable chunks.
Time Management Strategies
- Prioritize tasks based on importance and urgency.
- Use a planner or calendar to schedule study sessions and deadlines.
- Take regular breaks to prevent burnout and improve focus.
Stress Reduction Techniques
- Practice relaxation techniques such as deep breathing or meditation.
- Engage in physical activity to release tension.
- Seek support from friends, family, or a counselor if needed.
Motivational Quotes or Affirmations, Calc 3 exam 1 cheat sheet
- “The only way to do great work is to love what you do.” – Steve Jobs
- “Believe you can and you’re halfway there.” – Theodore Roosevelt
- “Don’t let fear or doubt hold you back. Go for it and make your dreams a reality.” – Unknown
FAQ Insights
Can I use this cheat sheet during the exam?
It’s generally not permitted to use external materials during exams. Please refer to your instructor’s guidelines for specific regulations.
How can I maximize the benefits of this cheat sheet?
Use it as a study companion to reinforce concepts, practice problem-solving, and identify areas where you need additional support.
Is this cheat sheet suitable for all levels of Calc 3 students?
This cheat sheet is designed to cater to students of varying proficiency levels. It provides a solid foundation for beginners and serves as a helpful refresher for more advanced students.

